DeltaVideo

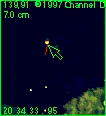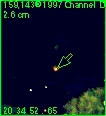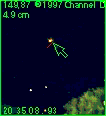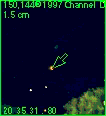
The STATIC IMAGE, above, shows an overlay of the movements of a nocturnal garden spider (whose motion was measured, tracked, and recorded by DeltaVideo, version 1.0.1) on a single video frame. The "web" is not an image of the actual web, but a vector trace generated by plotting the coordinates recorded by DeltaVideo, as the spider built the "wheels" (the "spokes" had already been constructed). The background is the first frame in the series, captured by DeltaVideo (the arrow indicates the spider position at the start of the recording). The other bright spots are lights in the background. The video was recorded with an inexpensive serial input video camera, at a resolution of 320 x 240, on a PowerBook 5300ce.

At the video camera's frame rate, a time-lapse sequence would have required nearly 7400 frames. DeltaVideo only saved the important parts of the sequence, and also quantitatively tracked the spider's motion in space and time, despite the spider's relatively small size with respect to the video frame.

Incidentally, the width of the entire web was approximately three feet (s/he was a very ambitious spider!) We also determined (from the log file) how far the spider travelled during the construction of the web: 45 meters (nearly half the way down a football field), at an average velocity of 1.7 cm/s.

• DeltaVideo can calculate an object's velocity by using the motion tracking coordinates, in conjunction with the time recorded for captured frames, and the user-supplied real-space frame size and measurement units. The velocity can be displayed on the thumbnail images, in a movie, or saved to a log file. The velocity calculations properly ignore periods when motion is not detected. However, the mean velocity (including idle periods) can be exported to the text log file.
• The object's motion vector can be included in the thumbnail display, or when creating a movie from the DeltaVideo capture file. An example of the motion vector is shown above, in the thumbnail viewer, and the frame from the extracted (and cropped) QuickTime movie. The motion vector is a linear trace drawn from the previous coordinate location(s).
• The peak velocity, interframe distance travelled, and cumulative distance travelled also can be included in the log file.

The motion vector is shown on the thumbnail images above (red curve), and the motion vector relative to the previous movie frame (the red "tail" extending from the tip of the motion tracking indicator arrow in the movie frame). The colors of stamped text, arrow (frame and fill), and motion vector all are adjustable.

The ANIMATION, below, is an x vs. y vs. time plot of the spider's motion, highlighting the time (z) axis. This shows the spatial movement of the spider with respect to time, over a period of 88.5 minutes.

The outlying points above the "neck" of the 3-D "funnel" indicate the spider greeting its second "visitor" of the evening. (See if you can spot the first. Hint: it's below the plane of rotation, protruding just before the funnel points "at" or "away from" you.) From the time axis, we can determine that this event occurred as the spider was completing construction of the web, and that the spider moved to that position and back to the center of the web in less than 0.72 seconds (the "dead" time of the video camera at the ambient illumination level) - intermediate motions were not recorded. In daylight, the same PowerBook / camera / DeltaVideo combination could have resolved movements as fast as 0.2 seconds (5 frames per second).

Copyright 1997, Channel D

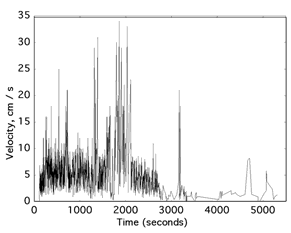
Shown above is a plot of the spider's peak velocity as a function of time. Again, the velocity was determined from the motion tracking information recorded in the DeltaVideo capture file, and extracted to a log file.

We also have QuickTime movies, extracted by DeltaVideo from the same file: Cinepak compressed, consisting of approximately 1100 captured frames. They have been cropped from the original frame size of 320 x 240 (half frame NTSC), and only include the motion detection "hot spot." The movie also was rendered at 50% size (to reduce download time). Cropping to the "hot spot" and specifying the final movie rendering scale are among DeltaVideo's options for extracting QuickTime movies from capture files.

The movies also use the brightness and contrast enhancement, averaged background, text stamping, coordinate tracking and animation options of DeltaVideo for extracting QuickTime movies.

The grayscale version (1 MB) just shows the spider and motion tracking indicator arrow. The full-color version (3 MB, repeating movie clip shown above) includes the motion tracking arrow, its coordinates, the spider's velocity (cm/s; seconds are implied in the display), capture time (resolved to 1/100 s), and motion tracking vector (the red "tail").

Note: the coordinate system shown in the QuickTime movie files uses the "computer graphics coordinate" convention [begun with the original Apple II, over 20 years ago (!)], which places the origin at the top left corner of the frame. The y - axis coordinates exported to the text log file also include the usual Cartesian 1st quadrant origin (bottom left hand corner). [y (Cartesian) = y (frame maximum) - y (computer graphics coordinate).]